14.1  NATIONAL WEATHER SERVICE

[Army Corps of Engineers]   [Natural Resources Conservation Service]   [Bureau of Reclamation]   [Geological Survey]   [Questions]   [Problems]   [References]   •   [Top]

Probable Maximum Precipitation

The Probable Maximum Precipitation (PMP) is the theoretically greatest depth of precipitation for a given duration that is physically possible over a given size storm area, at a particular geographical location, at a certain time of the year [7]. In view of the incomplete knowledge of meteorological processes, calculated PMP values are regarded as estimates [4]. For applications in hydraulic design, a suitable rainfall-runoff transform is used to convert the PMP into the Probable Maximum Flood (PMF).

Estimates of PMP are based on either statistical or deterministic approaches. The statistical approach is based on the analysis of time series. The deterministic approach, commonly referred to as the generalized estimate, is based on regional PMP isoline maps. In the United States, PMP determinations are commonly based on generalized estimates, using isoline maps developed by NOAA National Weather Service. The statistical approach is applicable in regions where a generalized estimate has not been developed.

Statistical Estimates of PMP. The statistical frequency formula is:

xT  =  x̄n + KT sn

(14-1)

in which x_T is the rainfall associated with a return period T, x̄_n and s_n are the mean and standard deviation of a series of n annual maxima, and K_T is the frequency factor associated with T (Chapter 6).

In terms of maximum rainfall, Eq. 14-1 can be expressed as follows:

xM  =  x̄n + KM sn

(14-2)

in which x_M is the maximum rainfall and K_M is the number of standard deviations that must be added to the mean in order to obtain x_M.

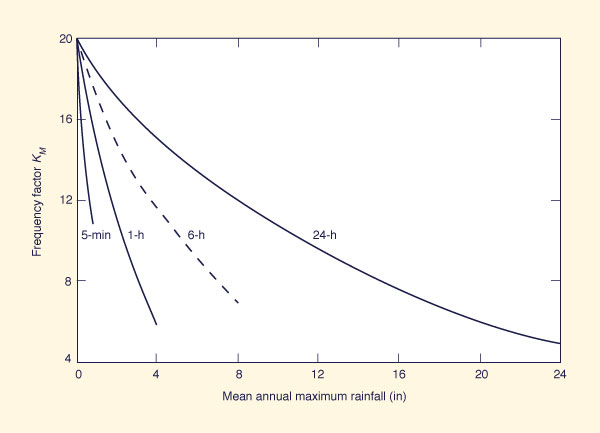
An empirical estimate of K_M may be obtained by enveloping a value of K_M based on a large number of computed values. The value K_M = 15 was considered initially by Hershfield [1] to be an appropriate enveloping value. This analysis was based on data from 2600 stations, approximately 90% of which were located in the United States. However, Hershfield's later studies [2] have shown that K_M actually varies with mean annual maximum rainfall and storm duration, as shown in Fig. 14-1. Note that this procedure gives point estimates of PMP; areal estimates may be obtained through the use of an appropriate depth-area relation.

Figure 14-1   Frequency factor KM as a function of mean annual maximum rainfall and storm duration [2].

The step-by-step procedure to estimate the D-hr PMP based on statistical data is the following:

1. Compile the D-hour annual-maxima rainfall series.

2. Calculate the mean and standard deviation of the D-hour annual-maxima rainfall series.

3. Using Fig. 14-1, determine the value of K_M as a function of mean annual maximum rainfall and D-hour duration.

4. Using Eq. 14-2, calculate the D-hour point PMP.

5. For basins in excess of 25 km², determine the areal PMP by reducing the point PMP using an appropriate depth-area relation.

Generalized Estimates of PMP. The procedure for determining generalized estimates of PMP varies from nonorographic to orographic regions. For nonorographic regions, the approach consists of performing three operations on observed areal storm precipitation: (1) moisture maximization, (2) transposition, and (3) envelopment. Moisture maximization consists of increasing the storm precipitation to a value that is consistent with the maximum moisture in the atmosphere, for the given storm's geographical location and month of occurrence.

Transposition involves the relocation of storm precipitation within a meteorologically homogeneous region, thereby increasing the quantity of data available for the evaluation of precipitation potential. Envelopment consists of the smooth interpolation between maxima for different durations and areas. In many cases, enveloping curves may increase the values of PMP obtained using only maximization and transposition. Envelopment also produces smooth geographical variations, assuring the consistency of mapped values.

For orographic regions, terrain plays an important role in precipitation, acting both to increase or reduce observed rainfall. Thus, orographic effects from storm-terrain interactions have a bearing on PMP determinations.

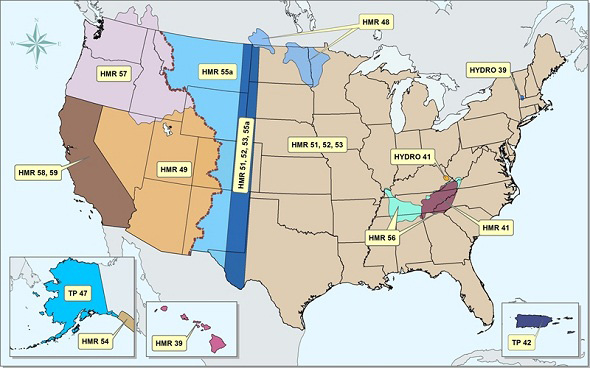
In the United States, the National Oceanic and Atmospheric Administration's (NOAA) National Weather Service (NWS) is responsible for the development of generalized estimates of probable maximum precipitation (PMP). The concepts and related methodologies are described in the NOAA NWS Hydrometeorological Report (HMR) series. Reports of current applicability are listed in Table 14-1. Figure 14-2 shows a map of the United States, depicting the regions covered by the HMR reports.

Table 14-1.  NOAA Hydrometeorological Reports (2015). (1) (2) (3) (4) Report No. Title Date Size (MB) HMR No. 39 Probable Maximum Precipitation in the Hawaiian Islands 1963 3.4 HMR No. 41 Probable Maximum and TVA Precipitation over the Tennessee River Basin above Chattanooga 1965 5.7 HMR No. 46 Probable Maximum Precipitation, Mekong River Basin 1970 16.2 HMR No. 48 Probable Maximum Precipitation and Snowmelt Criteria for Red River of the North above Pembina, and Sauris River above Minot, North Dakota 1973 1.6 HMR No. 49 Probable Maximum Precipitation Estimates, Colorado River and Great Basin Drainages 1977 6.2 HMR No. 51 Probable Maximum Precipitation Estimates, United States East of the 105th Meridian 1978 3.5 HMR No. 52 Application of Probable Maximum Precipitation Estimates, United States East of the 105th Meridian 1982 7.8 HMR No. 53 Seasonal Variation of 10-Square-Mile Probable Maximum Precipitation Estimates, United States East of the 105th Meridian 1980 3.3 HMR No. 54 Probable Maximum Precipitation and Snowmelt Criteria for Southeast Alaska 1983 4.6 HMR No. 55A Probable Maximum Precipitation Estimates - United States Between the Continental Divide and the 103rd Meridian 1988 103.5 HMR No. 56 Probable Maximum and TVA Precipitation Estimates with Areal Distribution for Tennessee River Drainages Less Than 3000 Mi2 in Area 1986 8.5 HMR No. 57 Probable Maximum Precipitation Estimates - Pacific Northwest States. Columbia River (including portions of Canada), Snake River and Pacific Coastal Drainages 1994 28.3 HMR No. 58 Probable Maximum Precipitation for California - Calculation Procedures 1998 10.9 HMR No. 59 Probable Maximum Precipitation for California 1999 21.4 HYDRO 39 Probable Maximum Precipitation for the Upper Deerfield River Drainage, Massachussets/Vermont 1984 1.9 HYDRO 41 Probable Maximum Precipitation Estimates for the Drainage Above Dewey Dam, Johns Creek, Kentucky 1985 1.4 T.P. No. 42 Generalized Estimates of Probable Maximum Precipitation and Rainfall-Frequency Data for Puerto Rico and Virgin Islands 1961 4.2 T.P. No. 47 Probable Maximum Precipitation and Rainfall-Frequency Data for Alaska 1963 3.1 Note:  Column 1 links connect to NOAA servers; Col. 2 links connect to local servers.

NOAA National Weather Service Figure 14-2   Regions covered by HMR reports (Click on the figure to enlarge).

Figure 14-1 shows the following:

• HMR 49 applies for the Colorado River and Great Basin drainages.

• HMR 51, 52 and 53 apply for regions east of the 105th meridian, i.e., the U.S. East and Midwest, including the central states.

• HMR 55A applies for the United States between the Continental Divide and the 103rd meridian (Note the overlap with HMR 51, 52, and 53).

• HMR 57 applies for the Pacific Northwest states and Pacific Coastal drainages.

• HMR 58 and 59 apply for the state of California.

• Other HMR reports apply for smaller regional areas, including Hawaii, Alaska, and Puerto Rico.

PMP Estimates for Regions East of the 105th Meridian. Procedures for estimating PMP in U.S. locations east of the 105th meridian are described in HMR 51, 52 and 53 [4, 5, 6]. HMR 51 contains a total of thirty (30) maps, corresponding to all possible combinations of five (5) durations (6, 12, 24, 48, and 72 h) and six (6) basin areas (10, 200, 1000, 5000, 10,000 and 20,000 mi²). The PMP map corresponding to the 24-h 10-mi² duration is shown in Fig. 14-3. A complete set of thirty (30) PMP maps applicable to U.S. regions east of the 105th meridian is shown in HMR 51 all-season PMP maps [4].

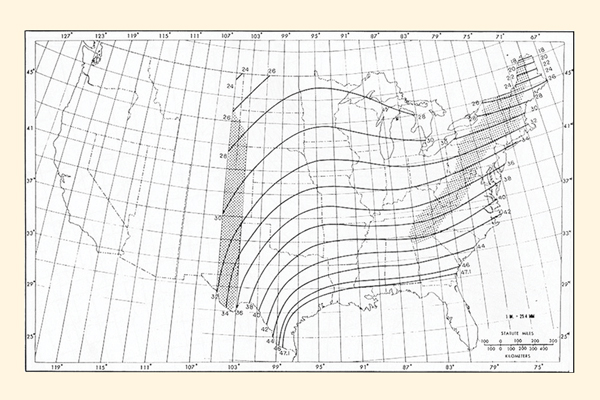
Figure 14-3   All-season PMP (in.) for 24-h 10-mi2 rainfall (Click on the figure to enlarge) [4].

Generalized PMP estimates can be obtained by the following steps, as explained in HMR 51 [4]:

1. Determine the geographical location and area of the drainage basin under study.

2. Using the maps in HMR 51, prepare a table of PMP depths applicable to the given geographical location. Use the maps of all five (5) durations and of at least four (4) areas comprising the drainage basin size of interest. For instance, for a basin size of 11,300 mi², tabulate 5 × 4 = 20 PMP depths (obtained from the 6-, 12-, 24-, 48-, and 72-hr maps, and from the 1000-, 5000-, 10,000-, and 20,000-mi² area maps).

3. For each of five (5) durations (6-, 12-, 24-, 48-, and 72-hr), plot PMP depths versus basin area on semilogarithmic paper, with PMP depth in the abscissas (arithmetic scale) and basin area in the ordinates (logarithmic scale), to obtain a PMP depth-area-duration (DAD) relation.

4. Using the PMP DAD relation developed in step 3, determine, for the given basin area, the applicable PMP depth for each of five (5) durations.

5. Plot PMP depth versus duration on arithmetic scales and draw a smooth curve connecting these points, to determine the PMP depth-duration relation for the given basin area size and geographical location.

6. Determine the 6-h incremental PMP depths using the PMP depth-duration relation developed in step 5. The 6-h incremental PMP is the PMP expressed in 6-h increments. This is accomplished by calculating the PMP depth for successive durations in multiples of 6 h, and subtracting two consecutive PMP depths to determine an incremental PMP value. For instance, the difference between the 24- and 18-h PMP depth is the 6-h incremental PMP depth associated with the 18- to 24-h time interval.

The application of HMR 51 to specific drainage basins, including the temporal and spatial distribution of the probable maximum storm is described in HMR 52 [5].

PMP Estimates for Regions West of the Continental Divide. The procedures for estimating PMP for U.S. regions west of the Continental Divide are described in the following HMR reports:

• HMR 49:  Colorado River and Great Basin drainages [3],

• HMR 57:  Pacific Northwest States) [8], and

• HMR 58 and HMR 59:  California [9, 10].

Unlike the case of the U.S. regions east of the 105th meridian, in regions west of the Continental Divide, the local storm (thunderstorm) is not enveloped with general-storm depth-duration data.

The estimates of local-storm PMP comprise durations varying from 15 minutes to 6 hours, and drainage areas between 1 and 500 mi² (2.59 and 1,295 km²). Local-storm PMP is applicable to the warm season, between May and October.

PMP Estimates for California. The procedures for estimating PMP in California are described in HMR 58 and HMR 59 [9, 10]. Note that HMR 58 is contained in HMR 59. HMR 58 provides estimates of general-storm PMP for a given drainage area, for durations of 1 to 72 hours and for areas of 10 to 10,000 mi² (25.9 to 25,900 km²), applicable on an annual basis (all-season PMP) and for any month of the year (seasonal PMP).

In HMR 58, the local-storm PMP comprises durations varying from 15 minutes to 6 hours, and drainage areas between 1 and 500 mi² (2.59 and 1,295 km²). For basins less than 500 mi², it is necessary to calculate both general- and local-storm PMP. The larger of these estimates is the applicable PMP for the given basin.

General-Storm PMP Computation for California. The procedure for estimating a general-storm all-season PMP in California is the following:

1. Basin location

   • Find the geographical location of the basin of interest.

   • Evaluate the basin outline and its drainage area.

2. All-season PMP index estimate [10-mi² 24-h ]

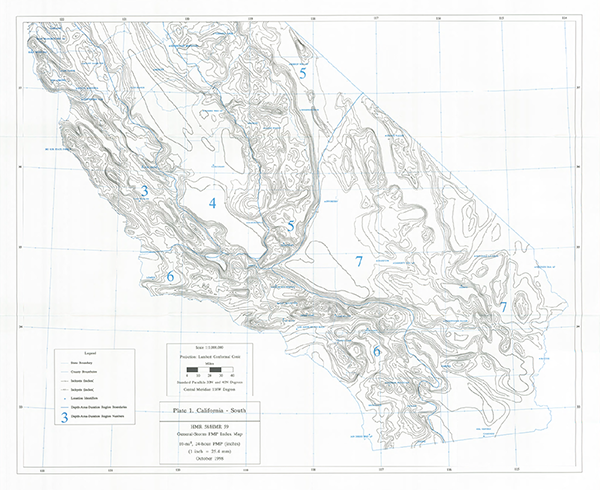
   • Overlay the drainage area on the appropriate general-storm PMP index map. Two maps are provided in HMR 59 [10]: California-South, also shown here as Fig. 14-4 (a); and California-North, also shown here as Fig. 14-4 (b).

   • Cover the entire drainage area with a uniform grid.

   • Determine the average general-storm PMP index [10-mi² 24-h] applicable to the entire drainage area.

3. Depth-duration ratios and 10-mi² PMP depths

   • Locate the basin within one of the regions of California shown in Fig. 14-5. Note that occasionally the basin may subtend more than one region.

   • Use Table 14-2 to determine the appropriate all-season PMP depth-duration ratios. Use proportionally region-weighted values if more than one subregion is subtended by the basin.

   • Multiply the depth-duration ratios (obtained in Step 3b) by the average general-storm PMP index [10-mi² 24-h] (obtained in Step 2c) to calculate 10-mi² PMP depths for durations of 1, 6, 12, 24, 48, and 72 h.

   Table 14-2.  California all-season PMP depth-duration ratios.*
   No.	Region	Duration (h)
   		1	6	12	24	12	24
   1	Northwest	0.10	0.40	0.73	1.00	1.49	1.77
   2	Northeast	0.16	0.52	0.69	1.00	1.40	1.55
   3	Midcoastal	0.13	0.45	0.74	1.00	1.45	1.70
   4	Central Valley	0.13	0.42	0.65	1.00	1.48	1.75
   5	Sierra	0.14	0.42	0.65	1.00	1.56	1.76
   6	Southwest	0.14	0.48	0.76	1.00	1.41	1.59
   7	Southeast	0.30	0.60	0.86	1.00	1.17	1.28
   * This table is the same as Table 2.1 of HMR 58.

4. Areal reduction factors

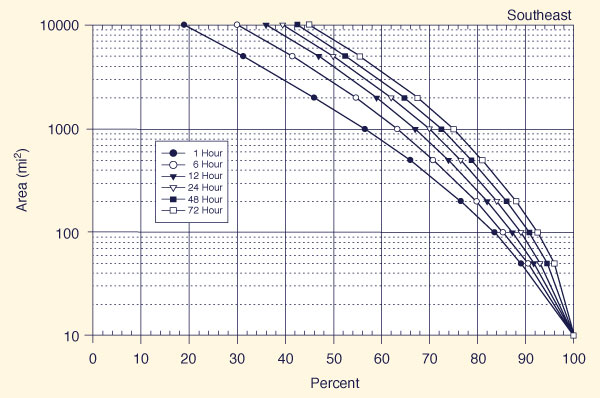
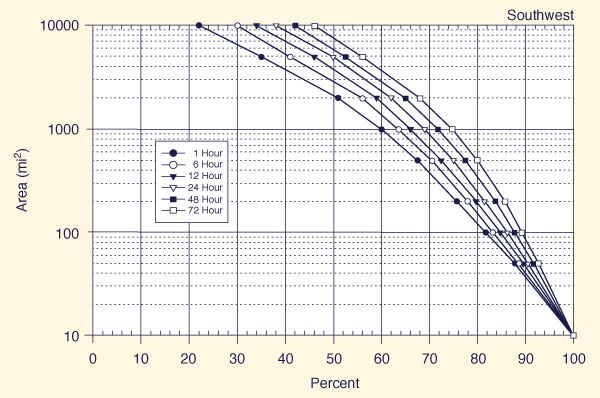
   • With the appropriate region known (Fig. 14-5), use one of Figs. 14-6 (a) to 14-6 (f) t obtain, for each of six (6) storm durations (1, 6, 12, 24, 48, and 72 h), the applicable all-season percent areal reduction.

   • Multiply the PMP depths (calculated in Step 3c) by the percent areal reductions (obtained in Step 4a) and divide by 100 to obtain the area-reduced PMP depths.

5. Incremental PMP values

   • Plot the results of Step 4b on an arithmetic graph and draw a smooth curve.

   • Obtain cumulative 6-h PMP values.

   • Each discrete 6-h PMP incremental value is the difference between two consecutive cumulative values.

6. Temporal distribution of PMP

   • To provide the most critical 72-h PMP storm sequence, group the four heaviest 6-h incremental values in a 24-h sequence, as follows:

     • Place the second highest value next to the highest, to provide the most critical 12-h sequence;

     • Place the third highest on either side of the 12-h sequence, to provide the most critical 18-h sequence, and

     • Place the fourth highest on either side of the 18-h sequence.

   • The 24-h heaviest sequence may be positioned anywhere in the 72-h period. The remaining eight 6-h amounts may be positioned anywhere else. For PMF purposes, critical PMP sequences are usually obtained by trial and error.

7. Spatial distribution of PMP

   • There are no fixed rules to develop a spatial storm pattern for general-storm PMP determinations. In the absence of local data, established NOAA National Weather Service isopercental curves may be used.

   • A spatial storm pattern for PMP may also be based on a significant storm with a sufficient number of observations. However, only a few California storms have sufficient detail to define a storm pattern.

Figure 14-4 (a)   General-storm PMP Index map for California - South (Click on the figure to display image) (Zoomable PDF file) [10].

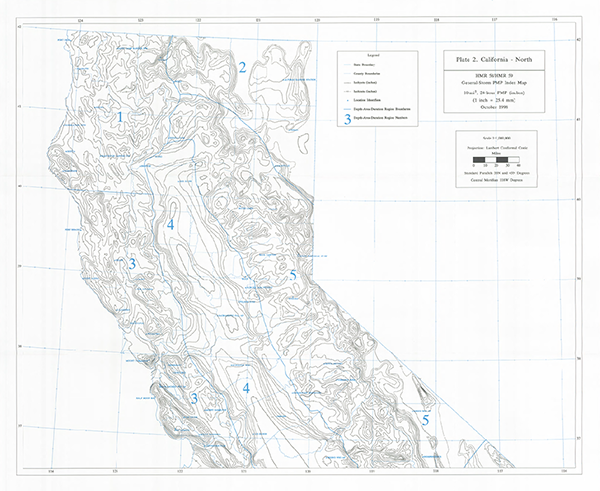
Figure 14-4 (b)   General-storm PMP Index map for California - North (Click on the figure to display image) (Zoomable PDF file) [10].

Figure 14-5   Regional boundaries for the development of depth-duration ratios in California (Click on the figure to enlarge) [9].

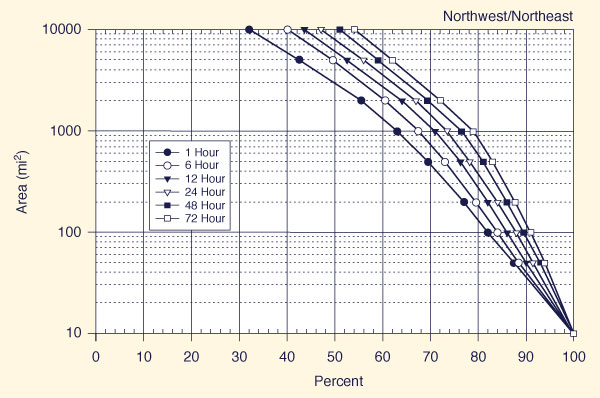
Figure 14-6 (a)   Depth-area-duration relation for the Northwest-Northeast region of California (Click on the figure to enlarge) [9].

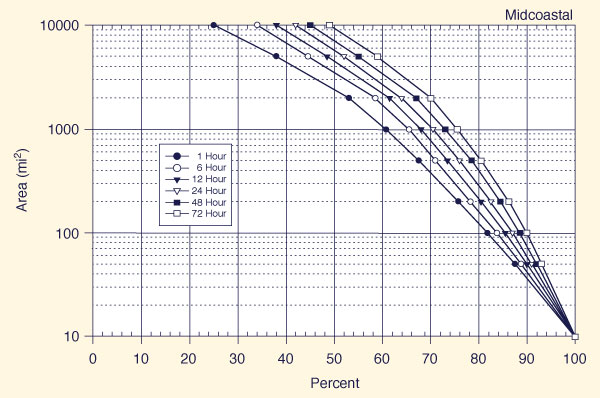
Figure 14-6 (b)   Depth-area-duration relation for the Midcoastal region of California (Click on the figure to enlarge) [9].

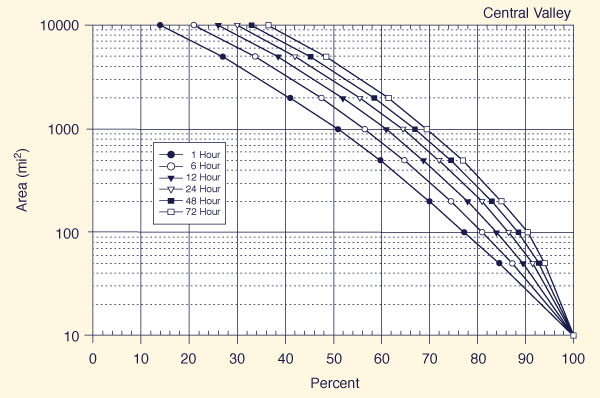
Figure 14-6 (c)   Depth-area-duration relation for the Central Valley region of California (Click on the figure to enlarge) [9].

Figure 14-6 (d)   Depth-area-duration relation for the Sierra region of California (Click on the figure to enlarge) [9].

Figure 14-6 (e)   Depth-area-duration relation for the Southeast region of California (Click on the figure to enlarge) [9].

Figure 14-6 (f)   Depth-area-duration relation for the Southwest region of California (Click on the figure to enlarge) [9].

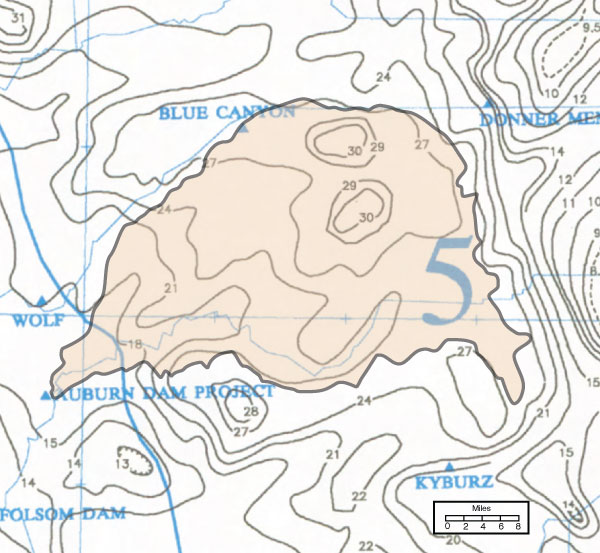
Example of General-Storm PMP Computation for California. The Auburn Dam drainage basin above Folsom Lake, California, is used as an example of a general-storm PMP computation. This example is described in HMR 58 [9] (Fig. 14-7).

Figure 14-7   Delineation of Auburn dam drainage basin on the general-storm PMP index map (Click on the figure to enlarge) [9].

The following steps are used to calculate the all-season PMP:

1. Basin location

   • The basin is located in Region 5 Sierra of California-North PMP index map, as shown in Fig. 14-5.

   • The drainage area is 973 mi².

2. All-season PMP index estimate [10-mi² 24-h]

   The average general-storm PMP index within the drainage area shown in Fig. 14-7 is calculated to be equal to 24.6 mi².

3. Depth-duration ratios and 10-mi² PMP depths

   The Auburn drainage basin is within Region 5 (Sierra), except for a very small portion near the damsite, which may be regarded as inconsequential (Fig. 14-5). Table 14-3 shows the applicable depth-duration ratios, extracted from Table 14-2.

   Table 14-3.  All-season PMP depth-duration ratios for the Sierra region.
   No.	Region	Duration (h)
   		1	6	12	24	12	24
   5	Sierra	0.14	0.42	0.65	1.00	1.56	1.76

   
   

   Table 14-4 shows the all-season 10-mi² PMP depths, obtained by multiplying the average [10 mi² 24-h] PMP index value of 24.6 in (obtained in Step 2) times each one of the depth-duration ratios shown in Table 14-3.

   Table 14-4.  All-season 10-mi2 PMP depths (in) for the Auburn drainage.
   No.	Region	Duration (h)
   		1	6	12	24	12	24
   5	Sierra	3.44	10.3	16.0	24.6	38.4	43.3

   
   

4. Areal reduction factors

   Use Fig. 14-6 (d) (Sierra) to determine all-season areal reduction factors for each of six (6) storm durations: 1, 6, 12, 24, 48, and 72 h. The areal reduction factors are shown in Table 14-5.

   Table 14-5.  All-season areal reduction factors for the Auburn drainage.
   Drainage area (mi2)	Duration (h)
   	1	6	12	24	12	24
   973	0.64	0.67	0.70	0.72	0.77	0.80

   
   

   Multiply the all-season 10-mi² PMP depths shown in Table 14-4 times the corresponding area reduction factors shown in Table 14-5 to obtain the all-season PMP depth-duration results shown in Table 14-6.

   
   

   Table 14-6.  All-season PMP depth-duration for the Auburn drainage.
   Duration (h)	1	6	12	24	12	24
   PMP depth (in)	2.2	6.9	11.2	17.7	29.6	34.6

   
   

5. Incremental PMP values

   Figure 14-8 shows depth-duration curves for the all-season PMP depths shown in Table 14-6. The All-season PMP is shown as a solid line. Also shown in the seasonal PMP (May), calculated in the seasonal PMP section (next section).

   Table 14-7 shows the 6-h cumulative all-season PMP depths obtained from Fig. 14-7. Table 14-8 shows the 6-h incremental all-season PMP depths obtained from Table 14-7.

   Table 14-7.  6-h cumulative all-season PMP depths for the Auburn drainage.
   Duration (h)	6	12	18	24	30	36	42	48	54	60	66	72
   PMP (in)	6.9	11.2	14.6	17.7	20.8	23.8	26.7	29.6	31.6	32.7	33.7	34.6

   
   

   Table 14-8.  6-h incremental all-season PMP depths for the Auburn drainage.
   Duration (h)	6	12	18	24	30	36	42	48	54	60	66	72
   PMP (in)	6.9	4.3	3.4	3.1	3.1	3.0	2.9	2.9	2.0	1.1	1.0	0.9

   
   

6. Temporal distribution of PMP

   Using the rules for temporal distribution of PMP, a possible 72-h storm sequence, in 6-h increments, is selected. Table 14-9 shows the chosen sequence.

   Table 14-9.  Possible all-season 72-h PMP storm for the Auburn drainage, in 6-h increments.
   Duration (h)	6	12	18	24	30	36	42	48	54	60	66	72
   PMP (in)	3.1	3.0	2.9	2.9	3.1	4.3	6.9	3.4	1.1	0.9	2.0	1.0

   
   

Figure 14-8   Depth-duration curves for the Auburn dam drainage basin (Click on the figure to enlarge) [9].

Example of Seasonal-Storm PMP Computation for California. The following example describes a seasonal-storm PMP computation for California. The procedure is for the month of May for the Auburn drainage (Fig. 14-7).

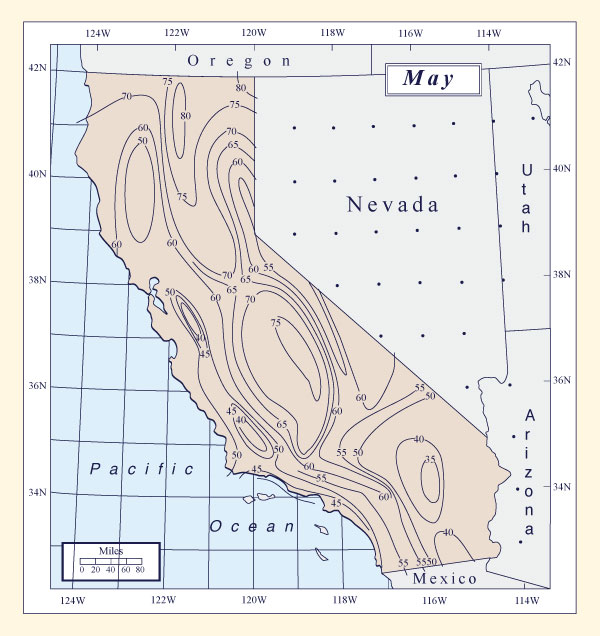
The all-season PMP index value, calculated in the previous section, is 24.6 in. Figure 14-9 shows the variation of general-storm PMP for the month of May, as a percent of all-season PMP. By placing the Auburn drainage (Fig. 14-7) in its geographical location in Fig. 14-9, a value of 68% for the Auburn drainage is obtained (See Note below). Therefore, the average PMP depth for the Auburn drainage for the month of May is: (68/100) × 24.6 = 16.7 in.

Note:  A complete set of 10-mi2 general-storm PMP for each month of the year, as a percent of all-season PMP, is given in General-storm monthly PMP maps.

Figure 14-9  10-mi2 24-h general-storm PMP for the month of May, as a percent of all-season PMP (Click on the figure to enlarge) [9].

Examination of the general-storm monthly PMP maps shows that the nearest all-season month for the Auburn drainage location is March, and the monthly offset is 2 (May to March, i.e., two-months difference). Table 14-10 shows the seasonally adjusted 2-month-offset 10-mi² PMP depth-duration ratios for the Sierra region.

Table 14-11 shows the seasonally adjusted May 10-mi² PMP, obtained by multiplying the calculated PMP depth for the month of May (16.7 in) by each one of the depth-duration ratios shown in Table 14-10.

Table 14-12 shows the seasonally adjusted areal reduction factors for the Sierra region, 2-month offset.

Table 14-13 shows the seasonally adjusted May PMP for the Auburn drainage, obtained by multiplying the seasonally adjusted May 10-mi² PMP values shown in Table 14-11 times the corresponding areal reduction factors shown in Table 14-12.

The seasonally adjusted May PMP depth-duration results shown in Table 14-13 are plotted in Fig. 14-8. Table 14-14 shows cumulative seasonally adjusted PMP depths at 6-h increments, obtained from the seasonal curve (May) shown in Fig. 14-8.

Table 14-15 shows the 6-h incremental seasonally adjusted (May) PMP depths obtained from Table 14-14.

Using the rules for temporal distribution of PMP, a possible 72-h storm sequence, in 6-h increments, is selected. Table 14-16 shows the chosen sequence.

Local-Storm PMP Computation for California. There are two procedures to calculate the local-storm PMP for California:

1. Average depth, and

2. Spatially distributed depth.

The PMP calculated using the average-depth method is usually greater than that calculated using the spatially distributed method.

The following steps are used to calculate the local-storm PMP:

1. 1-h 1-mi² local-storm PMP index

   • Determine a 1-h 1-mi² local-storm PMP index by overlaying the watershed of interest on the map shown in Fig. 14-10.

   • Use linear interpolation.

2. Mean basin elevation adjustment

   • Determine the mean basin elevation. No adjustment is necessary for elevations less than or equal to 6,000 ft.

   • For elevations greater than 6,000 ft, reduce the PMP index depth obtained in Step 1a by 9% for every 1,000 ft above the 6,000 ft threshold. For example, for the elevation of 8,700 ft, the adjustment is: [(8,700 - 6,000)/1000] × 9% = 24.3%, rounded to 24%. Therefore, the elevation-adjusted PMP is: PMP × (100 - 24)/100 = (0.76) PMP.

3. Storm duration adjustment

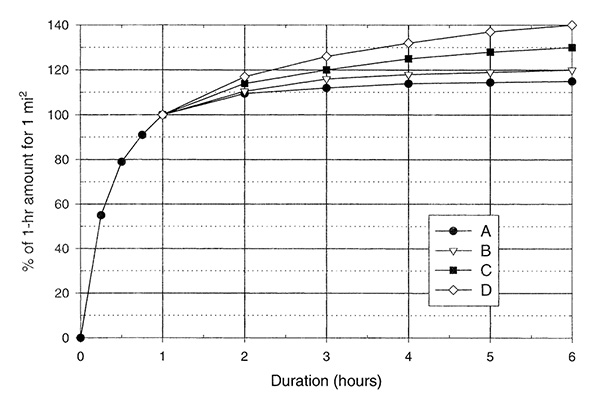
   • The 1-mi² local-storm PMP for durations less than 1 hour are obtained from Fig. 14-11 as a percentage of the 1-h 1-mi² local-storm PMP index obtained in Step 1a.

   • The 1-mi² local storm PMP for durations greater than 1 h is calculated as follows:

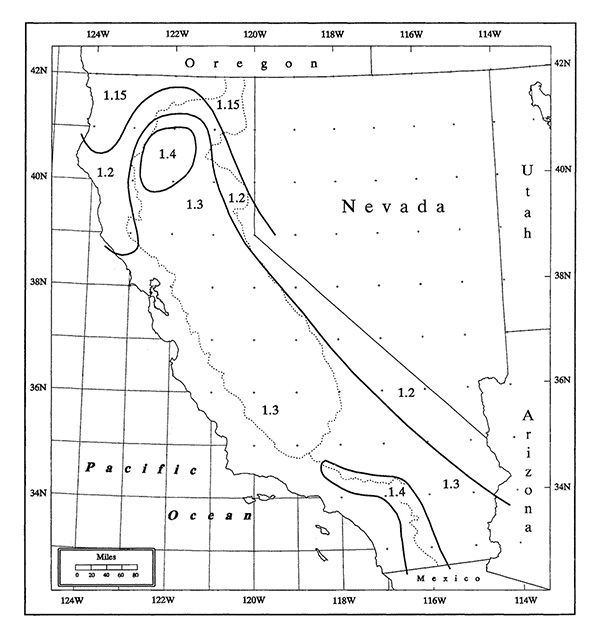
     • Locate the basin in Fig. 14-12 and determine the ratio of 6-h to 1-h local-storm PMP from the map, for example 1.15;

     • The values shown in Fig. 14-12 are labeled type curve A (1.15), B (1.2), C (1.3), or D (1.4);

     • Enter Fig. 14-11 for the chosen type curve (A, B, C, or D) to obtain the percentage of the 1-h 1-mi² local-storm PMP index for durations greater than 1 h, up to 6 h.

4. Areal reduction factors

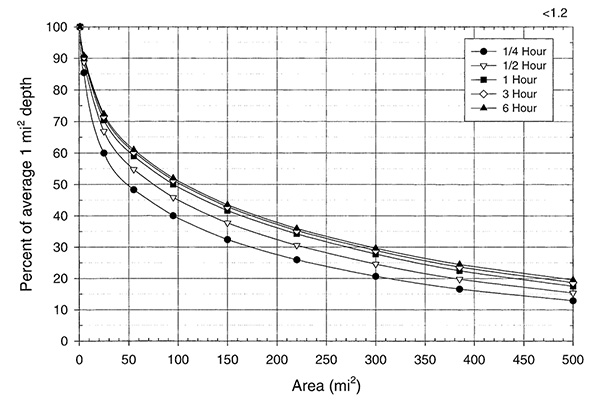
   Figure 14-13 shows the California 1-mi² local-storm depth-area relation for a 6-h to 1-h depth-duration adjustment ratio less than 1.2 (< 1.2). If this is the case, enter Fig. 14-13 with the basin area in the abscissas to the curve for the respective duration (1/4-h to 6-h) to determine the percent of 1-mi² depth in the ordinates.

   A complete set of California 1-mi² local-storm depth-area relations for all four values of 6-h to 1-h depth-duration adjustment ratios (< 1.2, 1.2, 1.3, and 1.4) is given in California local-storm depth-area relations.

5. Temporal storm distribution

   The review of local-storm temporal distributions for California shows that most storms have durations less than 6 h and that the greatest 1-h amount occurs in the first hour. The recommended sequence of hourly increments is as follows:

   1. Obtain the hourly increments by successive subtraction from the smoothed depth-duration curve (Fig. 4-10) or from Table 4-17.

   2. Arrange the hourly increments from largest to smallest: the most intense 1-h increment occurs in the first hour, the second most intense occurs in the second hour, and so forth.

   Table 14-17.  Depth-duration relations (in percent of 1-h amounts) applicable to 1-mi2 local-storm PMP in California.
   Duration (h)	A	B	C	D
   0	0	0	0	0
   1/4	55	55	55	55
   1/2	79	79	79	79
   3/4	91	91	91	91
   1	100	100	100	100
   2	109.5	110.5	114	117
   3	112	116	120	126
   4	114	118	125	132
   5	114.5	119	128	137
   6	115	120	130	140

6. Areal distribution of local-storm PMP

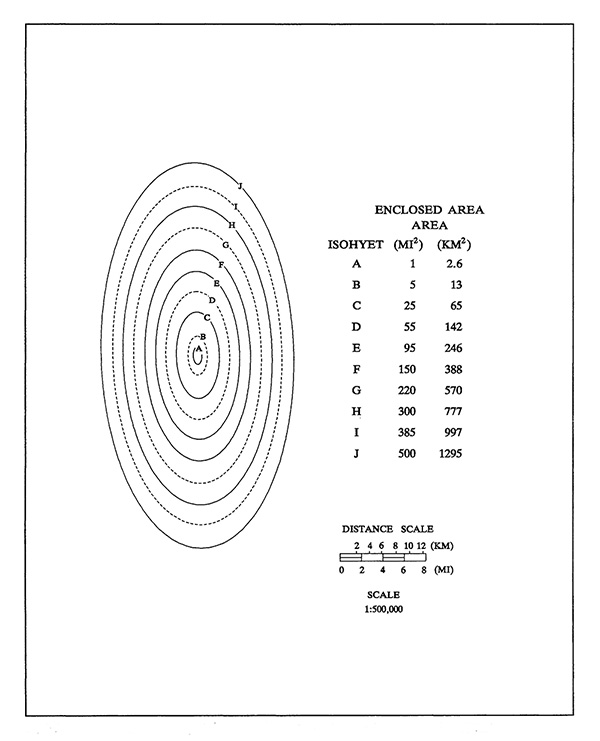
   Figure 4-14 shows an idealized isohyetal pattern for local-storm PMP areas up to 500-mi². The elliptical lettered pattern (A-J) of Fig. 4-13 and the tabulated percentages in HMR 58 Table 2.11 (ratio < 1.2), Table 2.12 (ratio = 1.2), Table 2.13 (ratio = 1.3), and Table 2.14 (ratio = 1.4) are used to describe the areal distribution of precipitation of a local-storm PMP. [These HMR 58 tables are given in HMR 58 local-storm areal distribution tables].

   • The 2:1 ratio of the major to minor axis of Fig. 14-14 should be placed only on a map at 1:500,000 scale.

   • The average index value from Step 2a or 2b is multiplied times each of the percentages from the appropriate HRM 58 local-storm areal distribution tables to obtain the value for each lettered isohyet (A-J).

   • Once the labels have been determined for each application, the pattern can be moved to different placements on the basin.

   • In most instance, the greatest volume of precipitation will be obtained when the pattern is centered in the drainage. However, peak flows may actually occur with placements closer to the drainage outlet.

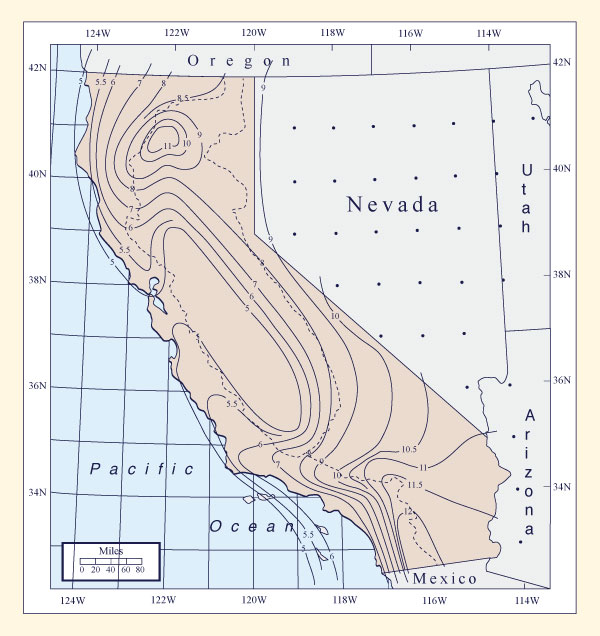
Figure 14-10   California 1-h 1-mi2 local-storm PMP index map (Click on the figure to enlarge) [9].

Figure 14-11   California 1-mi2 local-storm depth-duration relations (Click on the figure to enlarge) [9].

Figure 14-12   California 1-mi2 local-storm depth-duration adjustment ratios (Click on the figure to enlarge) [9].

Figure 14-13   California 1-mi2 local-storm depth-area relation for a 6-h to 1-h depth-duration adjustment ratio less than 1.2 (Click on the figure to enlarge) [9].

Figure 14-14   Idealized isohyetal pattern for local-storm PMP for areas up to 500-mi2 (Click on the figure to enlarge) [9].

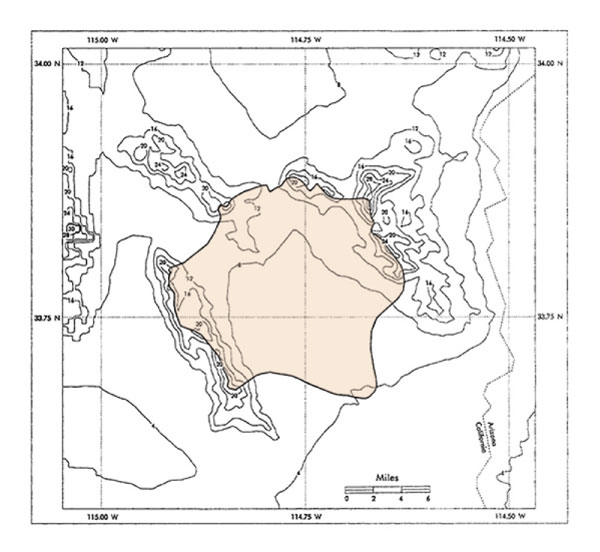
Example of Local-Storm PMP Computation for California. An example of local-storm PMP computation is described here. The selected basin is McCoy Wash, a small 176-mi² drainage located in southeastern California. This example is taken from HMR 58 [9].

Figure 14-15 shows the geographical location of the basin. Superposition of the basin outline on Fig. 14-10 results in the local-storm PMP index = 11.4 in. Figure 14-15 shows that the mean elevation of the basin is about 800 ft, well below 6,000 ft; therefore, no elevation adjustment is necessary, and the local-storm PMP index remains 11.4 in.

Figure 14-15   Drainage area of McCoy Wash, in Southeastern California, with elevation contours in hundredths of feet (Click on the figure to enlarge) [9].

From Fig. 14-12, the applicable adjustment ratio for the given geographical location is 1.3 (curve C in Fig. 14-11). Table 14-18 shows the depth-duration adjustment ratios and the corresponding local-storm 1-mi² PMP values adjusted for duration.

Figure 2-27 of HMR 58 (California local-storm depth-area relations) shows the depth-area relations for a California local-storm PMP for a 1-mi² 6-h to 1-h depth-duration adjustment ratio equal to 1.3 (curve C).

Table 14-19 shows the area-reduced 167-mi² local-storm PMP depth-duration for the McCoy Wash drainage. For each duration, the PMP values shown in Table 14-19 were obtained by multiplying the corresponding PMP value of Table 14-18 (for instance, 11.4 for 1 h) times the (percent of 1-mi²)/100 (that is, 0.43 for 1 h) shown in Table 14-19. Thus, for 1-h duration, the local-storm 167-mi² PMP is: 11.4 × 0.43 = 4.9 in. The results of Table 14-19 are shown in Fig. 14-16, and a smooth curve is fitted through the points.

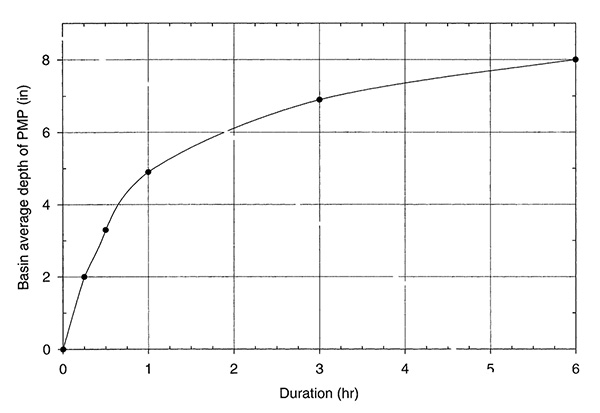
Figure 14-16   Average depth of local-storm PMP for the 167-mi2 McCoy Wash, California (Click on the figure to enlarge) [9].

Table 14-20 shows the 167-mi² local-storm PMP for the McCoy Wash drainage. The cumulative PMP values are obtained from Fig. 14-16 at every hourly interval. The incremental PMP values are obtained by successive subtraction of the respective cumulative values. The highest-to-lowest increment sequence shown in Table 14-20 is the recommended one for the local-storm PMP.

The areal distribution of PMP isohyets is shown in Fig. 14-14. This elliptical lettered pattern (A-J) comprises an area of 500 mi², at a scale of 1:500,000. For this example (McCoy Wash), the percentages from Table 2.13 of HMR 58 apply, i.e., for a basin with a 6-h to 1-h ratio of 1.3 (curve C). For completeness, Table 2-13 of HMR 58 is repeated here as Table 14-21.

Table 14-22 shows the isohyetal label values for local-storm PMP for the McCoy drainage. Values in this table were obtained by multiplying 11.4 in, corresponding to the 1-mi² 1-h PMP depth, times each one of the values of Table 14-21.

For comparison, the spatially averaged 6-h PMP for the 167-mi² McCoy Wash is 8 in (Table 14-19). On the other hand, from Table 14-22, for a 6-h PMP, the isohyetal labels range from 14.82 inches (tag A) enclosing 1 mi², to 4.33 inches (tag G) enclosing 220 mi².

Note that the isohyetal storm pattern of Fig. 14-14 will produce the spatially averaged PMP depth only if the basin under consideration is of elliptical shape, with a 2:1 ratio of the major to minor axis, and the ellipses are centered in a "perfect" drainage. When placed on an irregularly shaped drainage, the isohyetal storm pattern of Fig. 14-14 will produce an average depth which is less than the spatially averaged PMP depth. In this case, the spatially averaged PMP depth should be used, and the isohyetal pattern is interpreted as a guide to a possible spatial distribution of the local-storm PMP.

14.2  ARMY CORPS OF ENGINEERS

[Natural Resources Conservation Service]   [Bureau of Reclamation]   [Geological Survey]   [Questions]   [Problems]   [References]   •   [Top]   [National Weather Service]

The

14.3  NATURAL RESOURCES CONSERVATION SERVICE

[Bureau of Reclamation]   [Geological Survey]   [Questions]   [Problems]   [References]   •   [Top]   [National Weather Service]   [Army Corps of Engineers]

All

14.4  BUREAU OF RECLAMATION

[Geological Survey]   [Questions]   [Problems]   [References]   •   [Top]   [National Weather Service]   [Army Corps of Engineers]   [Natural Resources Conservation Service]

The

14.5  GEOLOGICAL SURVEY

[Questions]   [Problems]   [References]   •   [Top]   [National Weather Service]   [Army Corps of Engineers]   [Natural Resources Conservation Service]   [Bureau of Reclamation]

The

QUESTIONS

[Problems]   [References]  •   [Top]   [National Weather Service]   [Army Corps of Engineers]   [Natural Resources Conservation Service]   [Bureau of Reclamation]   [Geological Survey]

PROBLEMS

[References]   •   [Top]   [National Weather Service]   [Army Corps of Engineers]   [Natural Resources Conservation Service]   [Bureau of Reclamation]   [Geological Survey]   [Tennessee Valley Authority]   [Questions]

REFERENCES

•   [Top]   [National Weather Service]   [Army Corps of Engineers]   [Natural Resources Conservation Service]   [Bureau of Reclamation]   [Geological Survey]   [Questions]   [Problems]

1. Hershfield, D. M. (1961). "Estimating the Probable Maximum Precipitation." Journal of the Hydraulics Division, ASCE, Vol. 87, No. HY5, September, 99-116.

2. Hershfield, D. M. (1965). "Method for Estimating Probable Maximum Precipitation." Journal of the American Waterworks Association, Vol. 57, No. 8, August, 965-972.

3. NOAA Hydrometeorological Report No. 49. (1984). "Probable Maximum Precipitation Estimates, Colorado River and Great Basin Drainages." Silver Spring, Maryland, 176 p.

4. NOAA Hydrometeorological Report No. 51. (1978). "Probable Maximum Precipitation Estimates, United States East of the 105th Meridian." Washington, DC, June, 100 p.

5. NOAA Hydrometeorological Report No. 52. (1982). "Application of Probable Maximum Precipitation Estimates - United States East of the 105th Meridian." Washington, DC, August, 182 p.

6. NOAA Hydrometeorological Report No. 53. (1980). "Seasonal Variation of 10-mi² Probable Maximum Precipitation Estimates, United States East of the 105th Meridian." Silver Spring, Maryland, April, 96 p.

7. NOAA Hydrometeorological Report No. 55A. (1988). "Probable Maximum Precipitation Estimates, United States Between the Continental Divide an the 103rd Meridian." Silver Spring, Maryland, June, 280 p.

8. NOAA Hydrometeorological Report No. 57. (1994). "Probable Maximum Precipitation - Pacific Northwest States, Columbia River (including portions of Canada), Snake River, and Pacific Coastal Drainages." Silver Spring, Maryland, October, 353 p.

9. NOAA Hydrometeorological Report No. 58. (1998). "Probable Maximum Precipitation for California - Computational Procedures." Silver Spring, Maryland, October, 106 p.

10. NOAA Hydrometeorological Report No. 59. (1999). "Probable Maximum Precipitation for California." Silver Spring, Maryland, February, 419 p.