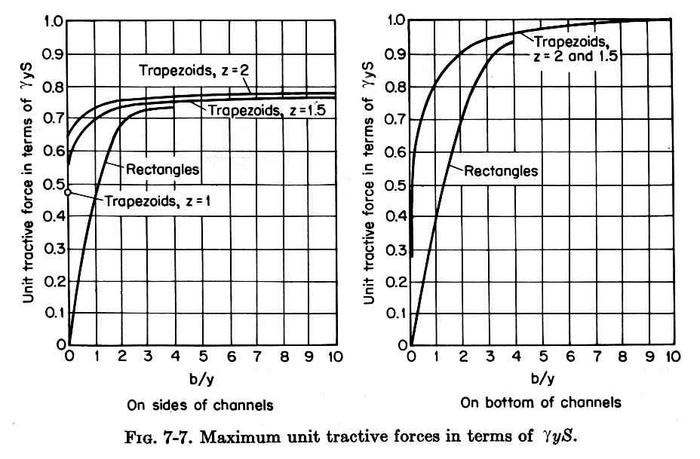
The acting shear stress on the sides:

T_s = 0.78 γ y S_o = (0.78) (62.4) (y) (0.00065) = 0.0316 y

Permissible tractive stress on level ground: τ_L = 0.3 lb/ft²

Permissible tractive stress on the sides: τ_s = K τ_L = (0.4472) (0.3) = 0.13416 lb/ft²

Under equilibrium: T_s = τ_s

Thus:

0.0316 y = 0.13416

From which flow depth y is:

y = 4.25 ft.

The Manning equation is:

Q = (1.486/n) A R^2/3 S^1/2 = (1.486/0.02) A (A^2/3/P^2/3) (S)^1/2

Q = (1.486/0.02) (A^5/3/P^2/3) (0.00065)^1/2

Q = 1.894 (A^5/3/P^2/3)

A = (b + zy) y = (b + 2y) y

P = b + 2 y (1+z²)^1/2 = b + 2 y (1+2²)^1/2 = b + 4.472 y

With y = 4.25 ft, try several values of b, until design Q is satisfied.

b	A	A5/3	P	P2/3	Q
60	291.12	12790	79.006	18.415	1315
40	206.12	7194	59.006	15.158	899
36	189.12	6232	55.006	14.465	816

Choose b = 36 ft; y = 4.25 ft; from which b/y = 8.5     still OK.

With b/y = 8.5, the coefficient of tractive force based on material on the bottom:    C_b = 0.99

The acting shear stress on level ground is:

T_L= 1.0 γ y S_o = (0.99) (62.4) (4.25) (0.00065) = 0.17 lb/ft²

T_L= 0.17 < 0.3 = τ_L

Therefore, the sides control the design.

b= 36 ft; y = 4.25 ft.     ANSWER.