The Saint-Venant equations describe the unsteady gradually varied flow in open channels.
- The flow is unsteady because discharge and flow area (and stage) vary in time and space.
- The flow is gradually varied because the assumption of hydrostatic pressure distribution is valid (vertical accelerations are negligible).
- The Saint Venant equations are a set of two (mass and momentum) nonlinear (actually quasi-linear)
partial differential equations describing
the movement of water in one dimension (x).
- The complete solution of the Saint-Venant equations has not been achieved, because of their nonlinear nature.
- Nonlinear PDE's cannot be solved completely with currently available methods of calculus.
- Simplifications are necessary, such as the assumption of linearity.
- A linear solution is convenient, but the nonlinearity is not accounted for.
- In the Saint-Venant equations, the nonlinearity is usually small (quasi-linearity).
- The Saint-Venant equations are:
- Water continuity:
|
u (∂d/∂x) + d (∂u/∂x) + (∂d/∂t) = 0
|
- Momentum:
|
(1/g) (∂u/∂t) + (u/g) (∂u/∂x) +
∂d/∂x + Sf - So = 0
|
- In the momentum equation, the term (1/g) (∂u/∂t) is the local acceleration slope.
- The term (u/g) (∂u/∂x) is the convective acceleration slope.
- The term ∂d/∂x is the slope due to the pressure-gradient.
- The term Sf is the friction slope.
- The term So is the bottom slope.
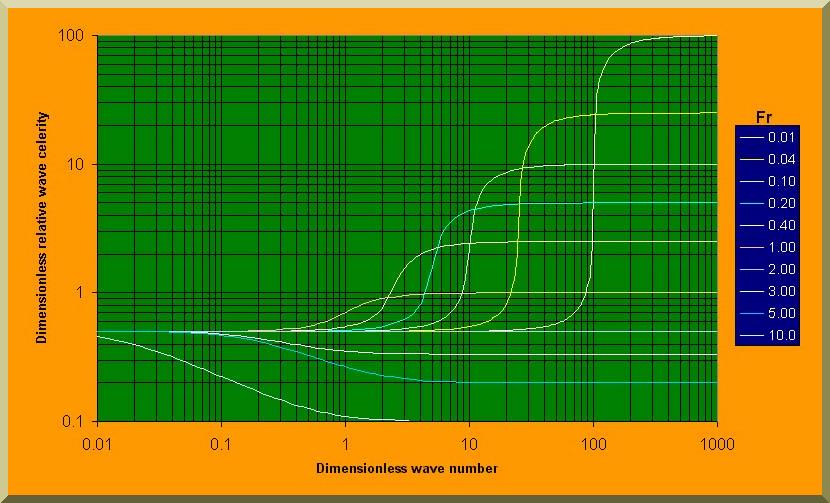
- The balance between these slopes (accelerations, or forces) determines the type of unsteady flow motion.
- The balance of friction and bottom slopes is called kinematic wave.
- The balance of local acceleration, convective acceleration, and pressure-gradient slopes is called
inertia-pressure wave.
- The balance of friction, bottom, and pressure-gradient slopes is called diffusion wave.
- The balance of all five (two acceleration, pressure-gradient, friction, and bottom) slopes is called a
dynamic wave.
- There are two types of acceleration because our frame of reference is fixed (Eulerian frame).
- If the frame of reference would be moving with the flow (Lagrangian frame),
there would only be one acceleration (Newton's).
|