CIVE 530 - OPEN-CHANNEL HYDRAULICS
LECTURE 5: UNIFORM FLOW

5.1 QUALIFICATIONS FOR UNIFORM FLOW

In uniform flow, the depth, area, velocity and discharge are constant.
In uniform flow, the slopes are all the same: friction, energy, water-surface, bottom.

S_f = S_e = S_w = S_o = S

There is no unsteady uniform flow; if the flow is unsteady, it is not uniform.
At high velocities, the flow becomes unstable and unsteady; this is the instability of uniform flow discussed by Chow.
Instability of the free surface leads to roll waves.
The Vedernikov number V determines the instability.
At V < 1, uniform (or equilibrium in natural channels) flow can occur.

5.2 ESTABLISHMENT OF UNIFORM FLOW

In uniform flow, gravitational forces are entirely balanced by frictional resistance.
"Nature likes uniform flow."
The depth of uniform flow is called "normal depth."
When y_n > y_c, this is normal subcritical flow.
When y_n < y_c, this is normal supercritical flow.

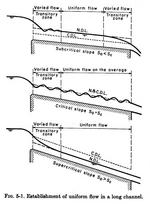
Fig. 5-1 (Chow)

5.3 VELOCITY OF UNIFORM FLOW

The velocity of uniform flow is:

v = C R^x S^y

C is a roughness or friction coefficient.
Values of x and y vary with roughness and cross-sectional shape.

5.4 CHEZY FORMULA

According to Chezy, the velocity of uniform flow is:

v = C R^1/2 S^1/2

where C = Chezy C.
The shear stress τ_b developed along the bottom is proportional to the square of the mean velocity v (this is the "quadratic resistance law"):

τ_b = ρ f v²

where f is a resistance (or drag) coefficient.

Fig. 5-2 (Chow)

The shear force along the wetted perimeter P of a control volume of length L is:

F_s = τ_b P L = ρ f v² P L

The body force (weight) resolved along the direction of motion is Wsinθ.

Assuming sinθ &asymp tan θ = S:

W sin θ = γ A L S

Therefore:

W sin θ = F_s

γ A L S = ρ f v² P L

γ A S = ρ f v² P

g R S = f v²

v = (g/f)^1/2 (RS)^1/2

C = (g/f)^1/2

f = g/C²

S = f v²/(gR)

S = f (D/R) [v²/(gD)]

For D ≈ R (hydraulically wide channel):

S = f [v²/(gD)]

S = f F²

5.6 MANNING FORMULA

According to Manning, the velocity of uniform flow in SI units is:

v = (1/n) R^2/3 S^1/2

where n = Manning's n.
The Manning formula was developed originally in SI units, and later converted to U.S. customary units.
The Manning formula in U.S. customary units is:

v = (1.486/n) R^2/3 S^1/2

The constant 1.486 comes from the unit conversion, and it is equal to 1/(0.3048)^1/3 = 1.4859
To compare with Chezy, the Manning formula is expressed as follows:

v = (1/n) R^1/6 R^1/2 S^1/2

Therefore:

C = (1/n) R^1/6

In the Manning formula, C is not a constant, but a function of R.
This implies that while C is not a constant, n is a constant.
However, n has been shown to vary in natural channels, with stage and discharge.
In natural rivers, n varies as a function of the flow regime: lower, transitional, or upper regime.
Lower regime has ripples and dunes, which are absent in upper regime.
Lower regime has skin and form friction; upper regime is mostly skin friction.

5.7 DETERMINATION OF MANNING ROUGHNESS

There is no exact method for selecting Manning roughness.
Recommendations:

Understand the factors that affect the value of n.
Consult a table of typical values.

Chow (B&W photos): minimum n = 0.008; maximum n = 0.250.
Barnes (color photos): minimum n = 0.024; maximum n = 0.075.
manningsn.sdsu.edu: minimum n = 0.024; maximum n = 0.075.
manningsn2.sdsu.edu: minimum n = 0.1; maximum n = 0.2.

Become acquainted with the appearance of typical channels whose n values are known.
Use theoretical velocity distributions.
Measure roughness directly (expensive, and stage-dependent).

5.8 FACTORS AFFECTING MANNING'S ROUGHNESS COEFFICIENT

The value of n is highly variable and depends on a number of factors.

A. Surface rougness

Fine grains result in a generally low value of n; coarse grains in a high value of n.

B. Vegetation

Vegetation retards the flow.
Maintenance of the channel determines the value of n.
Small depths require higher n.
Minimum n = 0.04 for drainage ditches cleared annually.
Use n = 0.05 if clearance is every two years.
Values greater than n = 0.1 may be warranted when channels are not cleared for several years.

C. Channel irregularities

Presence of sand bars, sand waves, ridges, depressions, and holes and humps in the channel bed.
The increase in n may be 0.005 or more.

D. Channel alignment

Smooth curvature with large radius will give a relatively low value of n.
Meandering increases n by about 30%.

E. Silting and scouring

Silting decreases n; scouring increases n.
Uneven deposits such as sand bars and sand waves are channel irregularities and will increase the roughness.

F. Obstructions

The presence of log jams and bridge piers tend to increase n.

G. Size and shape of channel

An increase in hydraulic radius may increase or decrease n, depending on the condition of the channel. There is no correlation between n and size and shape of channel.

H. Stage and discharge

The value of n varies with stage and discharge (See Ponce, page 274); first it decreases, then it increases, then it decreases again, as stages go from low flow to bankfull flow to overbank flow to very high flood stage.
n is a minimum at or somewhat above bankfull stage (This depends on the cross section).

I. Seasonal change

n increases in the growing season and decreases in the dormant season.

J. Suspended material and bedload

Suspended material and bedload consume energy and cause an increase in the apparent channel roughness.

Procedure for estimating the value of n:

n = (n₀ + n₁ + n₂ + n₃ + n₄) m₅

n₀ = basic n value for a straight, uniform, smooth channel
n₁ = value added to account for surface irregularities
n₂ = value added to account for variations in the shape and size of the cross section
n₃ = value added to account for obstructions
n₄ = value added to account for vegetation and flow conditions
m₅ = correction factor for channel meandering.

Table 5-5 (Chow)

Go to Chapter 6.

050927