CIV E 445 - APPLIED HYDROLOGY
SPRING 2014
HOMEWORK No. 7 SOLUTION
The calculations are shown in the following table.
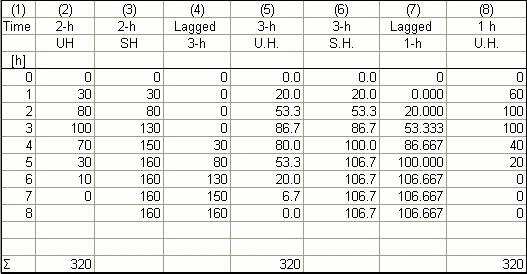
The 3-h unit hydrograph is shown in Col. 5; the 1-h unit hydrograph is shown in Col. 8. ANSWER.
The sum of Cols. 2, 5, and 8 is the same, verifying that all three hydrographs are unit hydrographs. The output from ONLINE S HYDROGRAPH verifies the hand calculations.
The total rainfall in the 6-h period is: P = 9 in. With runoff curve number CN = 84 and total rainfall P = 9 in., use Eq. 5-8 to calculate the direct runoff Q: Q = 7.06 in.
Assume φ-index between 0 and 0.5 in./h.
Therefore: [ (1.0 - φ) • 2 h + (2.0 - φ) • 2 h + (1.5 - φ) • 2 h ] = 7.06 in.
Solving for φ: φ = 0.323 in/h. Rainfall intensities and depths are as follows.
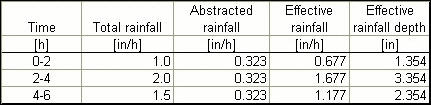
The unit hydrograph is convoluted with the effective rainfall depth pattern as shown in the following table.

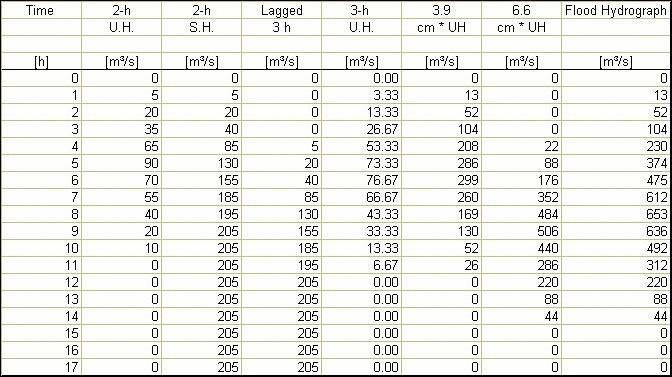
The composite hydrograph for the effective storm pattern is shown in Col. 6. ANSWER.
The output from ONLINE CONVOLUTION verifies the hand calculations.
To verify that the composite-hydrograph ordinates are correct, the radio of sums is: 3178 / 450 = 7.062. The sum of the effective rainfall depth is: 1.354 + 3.354 + 2.354 = 7.062. Therefore, the volumes under hyetograph and hydrograph are the same.
To convolute the 2-h unit hydrograph with the effective storm pattern defined at intervals of 3 h, it is necessary to change the unit hydrograph duration to 3 h. The change in unit hydrograph duration and unit hydrograph convolution are shown in the following table.
(1) For shallow concentrated flow, paved, use Fig. 5-18 with slope S = 0.01 to find the average velocity (along the hydraulic length) is: V = 2.05 ft/s = 0.625 m/s. Therefore, the time of concentration is: tc = L / V = 3350 / 0.625 = 5360 = 1.49 h.
(2) For 48% of the watershed area: for urban 1/3-ac lots, with lawns with 85% grass cover (i.e., in good hydrologic condition), 34% total impervious area, soil group C, use Fig 5-2(a) to find the pervious area (open space) CN: CN = 74. With pervious area CN = 74 and 34% total impervious area find the composite CN from Fig. 5-16: composite CN = 83.
(3) For 52% of the watershed area: for urban 1/3-ac lots, with lawns with 95% grass cover (i.e., in good hydrologic condition), 24% total impervious, 25% of it unconnected, soil group C, use Fig. 5-2(a) to find the pervious area (open space) CN: CN = 74. With pervious area CN = 74, 24% total impervious area, 25% of it unconnected, find the composite CN from Fig. 5-17: composite CN = 79.
(4) The runoff curve number for the entire watershed is obtained by areal weighing:
CN = [(83 × 48) + ( 79 × 52)] / 100 = 80.92. Use CN = 81.
(5) With CN = 81, use Eq. 5-49 to calculate the initial abstraction Ia: Ia = 1.2 cm
With P = 12 cm, the ratio Ia / P is: Ia / P = 0.1
With P = 12 cm, CN = 81, and R = 2.54, use Eq. 5-9 to find Q: Q = 6.97 cm.
Use Fig. 5-19(a), with rainfall type IA (Pacific Northwest region), time of concentration tc = 1.49 h, and ratio Ia / P = 0.1, to find the unit peak discharge qu: qu = 95 ft3 / (s-mi2 / in)
Converting to SI units: qu = 95 ft3 / (s-mi2 / in) × 0.0043 = 0.409 m3 / (s-km2-cm).
For 0.2% pond and swamp areas, use Table 5-12 to find F: F = 0.97.
Using Eq. 5-47: Qp = 0.409 m3 / (s-km2-cm) × 8.5 km2 × 6.97 cm × 0.97 = 23.5 m3/s.
The 50-y peak discharge is: Qp = 23.5 m3/s. ANSWER.
The output from ONLINE TR-55 verifies the hand calculations.