Problem 5-1

Since rainfall duration is greater than time of concentration, the flow is superconcentrated and the entire catchment is contrbuting. For subcatchments with different runoff coefficients, use a weighted formula for peak runoff (see Eq. 4-14): Q_p = I Σ(CA) =

Q_p = 55 mm/h • [ (0.3 • 175 • 30/100) + (0.5 • 175 • 40/100) + (0.9 • 175 • 30/100) ] ha • (10,000 m²/ha • 0.001 m/mm) / (3600 s/h) = Q_p = 14.97 m³/s.  ANSWER.

Problem 5-2

Several rainfall durations are tried, as shown in the following

tr (min)	I (mm/h)	Subarea A (C = 0.9) (ha)	Subarea B (C = 0.3) (ha)	Σ(CA)	Qp (m3/s)
20	147.7	66	51.33	74.8	30.68
30	123.9	66	77	82.5	28.4
40	107.5	66	102.67	90.2	26.95
50	95.5	66	128.33	97.9	25.96
60	86.1	66	154	105.6	25.26

The fraction of subarea B contributing to peak runoff increases linearly with rainfall duration.

Therefore: Q_p = I Σ(CA), in m³/s.

The 50-y peak runoff is the maximum value, corresponding to a 60-min duration: 30.68 m³/s.  ANSWER.

Problem 5-3

Using Eq. 4-19, the equilibrium outflow is:

q_e = iL/3600 = (40 mm/h • 100 m • 0.001 m/mm • 1000 L/m³) / (3600 s/h) =

q_e = 1.11 L/s/m = 1.11 • 10^-3 m³/s/m = 0.00111 m²/s.

For T = 20°C, ν = 1.0 • 10^-6 m²/s (Table A-1). Using Eq. 4-27: C_L = (9.81 m/s² • 0.015) / (3 • 1.0 • 10^-6 m²/s) = 49,050 m^-1s^-1.

In Eq. 4-25, for laminar flow, b = C_L, and m = 3. Therefore:

h_e = (q_e / C_L)^1/3 = (0.00111/49,050)^1/3 = 0.00283 m = 2.83 mm.  ANSWER.

For T = 30°C, ν = 0.801 • 10^-6 m²/s. Using Eq. 4-27: C_L = 61,236 m^-1s^-1. Therefore, with Eq. 4-25: h_e = 0.00263 m = 2.63 mm.  ANSWER.

Problem 5-4

The rainfall excess in m/s is:

i = (25 mm/h • 0.001 m/mm) / (3600 s/h) = 6.94•10^-6 m/s.

q_e = 6.94•10^-6 m/s • 80 m = 0.0005555 m³/s/m = 0.5555 L/s/m.

For 75% turbulent flow, m = 2. Therefore, in Eq. 4-29:

t_e = [ 2 • (0.05 • 80)^{1 / 2} ] / [(6.94•10^-6)^{1 / 2} • 0.01^{1 / 4}] = 4800 s.

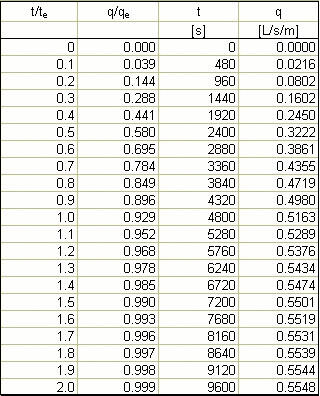
Using Eq. 4-36, the rising limb of the overland flow hydrograph is calculated as shown in the following table.  ANSWER.